Definition
A measure of the process capability (potential of the process to meet specifications), after taking into account the centering of the process. It is calculated by dividing the distance of the process mean to the nearest specification by 3 Standard Deviations of the process. The process must be in control before assessing Cpk. If the process is centered exactly in the middle of the tolerance, Cpk is exactly equal to the maximum capability (Cp).
Examples
Cpk is calculated as: Min((Mean-LSL)/3s, (USL-Mean)/3s)
Where USL and LSL are the upper and lower specification limits respectively, 'Mean' is the Process average and 's' is the process standard deviation estimated by dividing the average range (Rbar) of the subgroups by the appropriate SPC (statistical process control) constant, d2, which is based on the subgroup size. In other words, s = Rbar/d2.
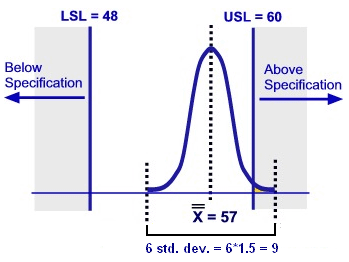
Consider the example used for the Cp calculations earlier:
The Lower Specification Limit is 48
The Upper Specification Limit is 60
Therefore, the Tolerance is 60 - 48, or 12
The std. dev is estimated to be 1.5
The process mean is 57
Then Cpk is the minimum of: (57-48)/4.5 = 2 and (60-57)/4.5 = 0.67.
So Cpk is 0.67.
Application
A Cpk less than 1.0 indicates that a part of the process output is defective. The Z value corresponding to this Cpk of 0.67 is 3(0.67) = 2. Since this Cpk represents the part of the process distribution that is above the USL, we find the area of the normal distribution above Z = 2, which is 0.023. Hence, the fraction defective of the process is 2.3%.
