Definition

The kurtosis value describes the peakedness of a distribution. Sharply peaked distributions have positive kurtosis (kurtosis > 0) and are called leptokurtic. Flatter than normal distributions have negative kurtosis (kurtosis < 0), also called platykurtic. A normal distribution has a kurtosis = 0 and it is called mesokurtic.

Examples

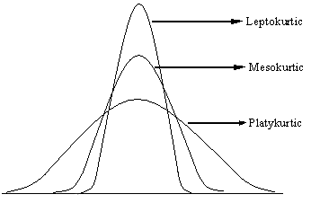
 Examples of Leptokurtic distribution:
Laplace
Logistic

Examples of Mesokurtic distribution:
Family of Normal distributions

Examples of Platykurtic distribution:
Uniform (continuous or discrete)

External Links

NIST Statistics Handbook: Measures of Skewness and Kertosis - http://www.itl.nist.gov/div898/handbook/eda/section3/eda35b.htm